Trigonometry is one of the topics that belongs to the quantitative aptitude section of the SSC CGL exam. It is considered one of the complex topics of the quant section. In SSC CGL Tier 1 exam, you can expect at least 2-3 questions on this topic. Most candidates make mistakes and leave the questions because they don’t know the right approach to study this topic. If you are also one of them then this blog is for you. In this blog, we have provided definitions, formulas, preparation tips, questions, and many other things by which you can take your preparation of trigonometry for SSC CGL to the next level. So go through this blog carefully and don’t forget to attempt our free SSC CGL mock test, topic-wise tests, and other free preparation resources.
Trigonometry for SSC CGL: Topic-wise and Complete Preparation
Solving the topic test is very important if you want to get in-depth knowledge of the individual topic. Below, we have provided the topic tests for each section, including quizzes for current affairs, previous year question papers, and full-length mock tests.
| Click For Mock Test – > | SSC CGL Free Full-Length Mock Test |
| Click For SSC CA – > | SSC CGL Free Weekly Current Affairs Tests |
| Click For Quant – > | Quantitative Aptitude Free Topic Tests |
| Click For Reasoning – > | General Reasoning Free Topic Tests |
| Click For English – > | English Comprehension Free Topic Tests |
| Click For SSC GA – > | General Awareness Free Topic Tests |
| Click For Sectional Tests -> | SSC CGL Free Sectional Mock Tests |
| Click For PYQs – > | Free SSC CGL Previous Year Question Papers |
Concept of Trigonometry: Definition, Formulas
Below we have provided the definition and formulas for trigonometry learn and understand the formulas.
Definition:
Trigonometry is a topic of quantitative aptitude that deals with the relationships between the angles and sides of triangles, particularly right-angled triangles. The fundamental concept in trigonometry revolves around the ratios of the sides of a right-angled triangle relative to its angles.
Basic Trigonometric Ratios:
In a right-angled triangle, let:
- θ be one of the non-right angles,
- Opposite be the side opposite to the angle θ,
- Adjacent be the side adjacent to the angle θ,
- Hypotenuse be the side opposite the right angle (the longest side).
The six fundamental trigonometric ratios are defined as:
1. Sine (sin):
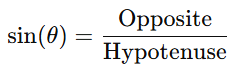
2. Cosine (cos):
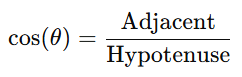
3. Tangent (tan):
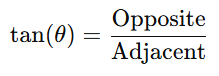
4. Cosecant (csc): (Reciprocal of sine)
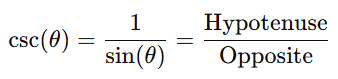
5. Secant (sec): (Reciprocal of cosine)
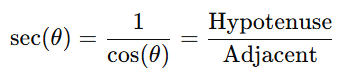
6. Cotangent (cot): (Reciprocal of tangent)
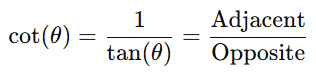
Pythagorean Theorem:
For a right-angled triangle, the relationship between the sides is given by:
Hypotenuse2=Opposite2+Adjacent2
This is a fundamental relationship used to derive many trigonometric identities.
Trigonometric Identities:
1. Pythagorean Identities:
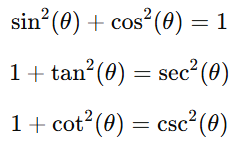
2. Reciprocal Identities:
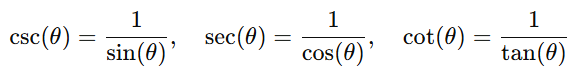
3. Quotient Identities:
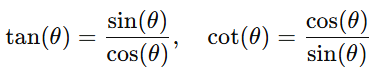
4. Co-function Identities:
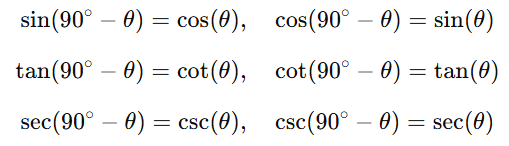
Angle Sum and Difference Identities:
1. Sine of sum and difference:
sin(A+B)=sin(A)cos(B)+cos(A)sin(B)
sin(A−B)=sin(A)cos(B)−cos(A)sin(B)
2. Cosine of sum and difference:
cos(A+B)=cos(A)cos(B)−sin(A)sin(B)
cos(A−B)=cos(A)cos(B)+sin(A)sin(B)
3. Tangent of sum and difference:
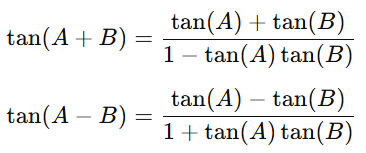
Double Angle and Half-Angle Formulas:
1. Sine Double Angle:
sin(2θ) = 2 sin(θ) cos(θ)
2. Cosine Double Angle:
cos(2θ) = cos2(θ)−sin2(θ)
or equivalently:
cos(2θ) = 2 cos2(θ) − 1
cos(2θ) = 1−2 sin2(θ)
3. Tangent Double Angle:
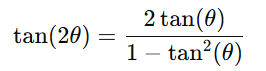
4. Half-Angle Formulas:
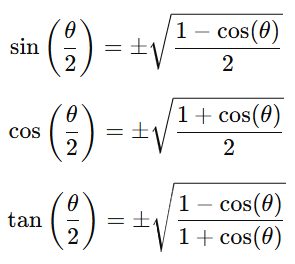
Law of Sines and Law of Cosines:
These laws are used in any triangle, not just right-angled ones.
1. Law of Sines:
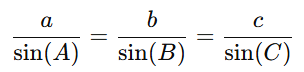
where a,b,c are the sides of the triangle, and A,B,C are the opposite angles.
2. Law of Cosines:
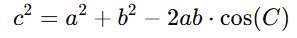
This formula helps find a side of a triangle when two sides and the included angle are known, or it can be used to find angles when all three sides are known.
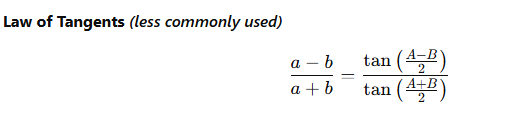
Trigonometric Functions of Any Angle:
For angles greater than 90°, the values of trigonometric functions can be determined using the unit circle. The unit circle approach defines each trigonometric function for all real angles (not just those in a right triangle).
Trigonometry Table
Below we have provided the trigonometry table for standard angles and some non-standard ones.
| Trigonometry Table | ||||||||
|---|---|---|---|---|---|---|---|---|
| θ | 0° (0 radians) | 30° (π/6) | 45° (π/4) | 60° (π/3) | 90° (π/2) | 180° (π) | 270° (3π/2) | 360° (2π) |
| sin | 0 | 1/2 | 1/√2 | √3/2 | 1 | 0 | -1 | 0 |
| cos | 1 | √3/2 | 1/√2 | 1/2 | 0 | -1 | 0 | 1 |
| tan | 0 | 1/√3 | 1 | √3 | ∞ | 0 | ∞ | 0 |
| csc | ∞ | 2 | √2 | 2/√3 | 1 | ∞ | -1 | ∞ |
| sec | 1 | 2/√3 | √2 | 2 | ∞ | -1 | ∞ | 1 |
| cot | ∞ | √3 | 1 | 1/√3 | 0 | ∞ | 0 | ∞ |
Trigonometry Formulas for SSC CGL PDF
There are so many trigonometry formulas for SSC CGL that you should prepare if you want to score good marks in this topic in the SSC CGL exam. For your convenience, we have provided all the above formulas in a PDF that you can download for free. You are advised to take a printout of the PDF after downloading it to prepare or learn formulas easily for the SSC CGL Tier 1 exam.
Questions of Trigonometry for SSC CGL, Download Free PDF
Below, we have provided the trigonometry questions for the SSC CGL exam. These questions were asked in the previous year’s question papers. By using these questions, you will be able to determine the type of trigonometry questions asked in the exam.
Question 1:
What is the value of sin(B – C) cos(A – D) + sin(A – B) cos(C – D) + sin(C – A) cos(B – D)?
(a) 3/2
(b) –3
(c) 1
(d) 0
Question 2:
If tan θ – cot θ= a and cos θ + sin θ= b, then (b2 –1)(a2 + 4) = ?
(a) 2
(b) 1
(c) 0
(d) 4
Question 3:
If sin θ + sin 2 θ = x and cos θ + cos 2 θ =y, then (x2 + y2)(x2 + y2 – 3) = ?
(a) 2y
(b) 3y
(c) 4y
(d) 5y
Question 4:
If cosecq – sinq =1 and secq – cosq = m, then l2 m2(l2 + m2 + 3) = ?
(a) 0
(b) 1
(c) –1
(d) 2
Question 5:
If tan A + sinA = p and tan A – sinA = q then which of the following is true?
(a) p2 + q2 = 4√pq
(b) p + q = pq
(c) p– q = pq
(d) p2– q2 = 4√pq
Question 6:
If 𝑐𝑜𝑠𝜃 + 𝑠𝑖𝑛𝜃 = m, 𝑠𝑒𝑐𝜃 + 𝑐𝑜𝑠𝑒𝑐𝜃 = n then n(m2 – 1) is equal to?
(a) 2m
(b) mn
(c) 4mn
(d) 2n
Question 7:
If a(tanθ + cotθ)=1, sinθ + cosθ = b and 0 < θ < 90 then relation between a and b?
(a) b2 = 2(a + 1)
(b) b2 = 2(a – 1)
(c) 2a = b2 – 1
(d) 2a = b2 + 1
Question 8:
If A is an acute angle and cot A + cosec A = 3, then the value of sin A is
(a) 1
(b) 4/5
(c) 3/5
(d) 0
Question 9:
If A + B + C = π, then cos2A + cos2B + cos2C = ?
(a) 1 + 4 cosA cosB cosC
(b) –1 + 4 sinA sinB cosC
(c) –1 – 4 sinA cosB sinC
(d) 1 + 4 sinA sinb cosC
Question 10:
If A + B + C = 180º, then sin2A + sin2B + sin2C = ?
(a) 4 sinA sinB cosC
(b) 4 cosA cosB cosC
(c) 4 sinA sinB sinC
(d) 8 sinA sinB sinC
Trigonometry Test for SSC CGL
As we know, solving trigonometry questions can take time, and SSC CGL is a time-bounded exam. It means you should have good speed, accuracy, and time management skills if you want to solve trigonometry questions correctly within the time range. For that, here we have provided the trigonometry test for SSC CGL, which gives you the feel of the real exam environment. The questions that we have provided in the test match the level of questions asked in the exam. So, click on the link given below and start your preparation for the exam.
Trigonometry SSC CGL Preparation Tips
Below we have provided some tips that will help you prepare for trigonometry for the SSC CGL exam. Read these tips carefully so that you become able to solve trigonometry questions for SSC CGL exam effectively.
- Understand Concepts and Formulas: There are many formulas, ratios, values, etc in trigonometry. So first learn them and understand them. It will help you to solve questions quickly and with accuracy.
- Learn Trigonometric Identities: Focus on mastering the key trigonometric identities, such as:
- Pythagorean identities: sin2𝜃 + cos2𝜃 = 1
- Angle sum and difference formulas.
- Double angle and half angle formulas.
- Reciprocal identities.
- Co-function identities.
- Use Shortcuts and Tricks (if applicable): it is possible that many questions can be solved by using tricks and shortcuts. You are advised to focus on those tricks and shortcuts so that you become able to solve those questions within the given time.
- Draw Diagrams and Visualize the Problems: For complex problems, trying to draw a diagram is possible because visualizing a problem in terms of a triangle or a unit circle can help you better understand the relationships between the angles and sides.
- Practice Questions as Much as Possible: At last, try to solve questions from easy to advanced levels as much as possible because the SSC can ask questions of any level. So you should be ready for any type of questions. You are advised to solve mock tests as much as possible so that you can practice trigonometry for SSC CGL on our website.
Join our exclusive Telegram group for expert guidance, personalized tips, and real-time solutions to boost your SSC exam prep. [Click here to join now!]
| Other Blogs of SSC CGL 2025 | |
| SSC CGL Notification | SSC CGL Syllabus |
| SSC CGL Study Plan | SSC CGL Exam Pattern |
| SSC CGL Cut Off | SSC CGL Preparation Strategy |
| SSC CGL Previous Year Question Papers | |
SSC CGL Trigonometry Preparation: FAQs
Trigonometry is a topic of quantitative aptitude that deals with the relationships between the angles and sides of triangles, particularly right-angled triangles. The fundamental concept in trigonometry revolves around the ratios of the sides of a right-angled triangle relative to its angles.
You can get the questions of trigonometry for SSC CGL exam in this blog.
You can get the trigonometry tests for SSC CGL exam, on PracticeMock website.
You can expect at least 2-3 questions from trigonometry for SSC CGL exam.
- Sign Up on Practicemock for Updated Current Affairs, Free Topic Tests and Free Mini Mocks
- Sign Up Here to Download Free Study Material
Free Mock Tests for the Upcoming Exams
- IBPS PO Free Mock Test
- RBI Grade B Free Mock Test
- IBPS SO Free Mock Test
- NABARD Grade A Free Mock Test
- SSC CGL Free Mock Test
- IBPS Clerk Free Mock Test
- IBPS RRB PO Free Mock Test
- IBPS RRB Clerk Free Mock Test
- RRB NTPC Free Mock Test
- SSC MTS Free Mock Test
- SSC Strenographer Free Mock Test
- GATE Mechanical Free Mock Test
- GATE Civil Free Mock Test
- RRB ALP Free Mock Test
- SSC CPO Free Mock Test
- AFCAT Free Mock Test
- SEBI Grade A Free Mock Test
- IFSCA Grade A Free Mock Test
- RRB JE Free Mock Test
- Free Banking Live Test
- Free SSC Live Test