SSC CHSL 2023 recruitment notification has been released featuring a total of 1600 vacancies. As per the notification the last date and time for receipt of online applications is 8th June 2023. So, we would like to suggest to the aspiring candidates not to wait for the last date and try filling the application forms as early as possible in order to avoid any last minute hassles. Those who are done with the online application process can attempt the SSC CHSL free mock test to know the kind of questions which are asked in the actual examination. Moreover, for those who have already started their exam preparation we have got for them SSC CHSL Quant Practice Questions for Free Preparation along with detailed solutions. Let’s first take a look at the exam pattern.
SSC CHSL Tier I Exam Pattern
| Tier | Part | Subject(Not in sequence) | Number of Questions/ Maximum Marks | Time Duration (For all four Parts) |
| I | I | English Language (Basic Knowledge) | 25/ 50 | 60 Minutes (80 Minutes for candidates eligible for scribe as per Para-7.1, 7.2 and 7.3) |
| II | General Intelligence | 25/ 50 | ||
| III | Quantitative Aptitude (Basic Arithmetic Skill) | 25/ 50 | ||
| IV | General Awareness | 25/ 50 |
SSC CHSL Quant Practice Questions for Free Preparation
Topic: SSC_ Averages
Level: 0
1.) The mean of five numbers is 18. If one number is excluded, their mean is 16. The excluded number is:
a.) 30
b.) 26
c.) 36
d.) 32
Answer: b)
Solution:
Sum of five numbers = 18 × 5 = 90
Sum when one number is excluded = 16 × 4 = 64
So, excluded number = 90 – 64 = 26
Hence, option b.
Topic: SSC_ Time, speed and distance
Level: 0
Attempt SSC CHSL Tier I 2023 Free Mock Test
2.) After seeing a policeman from a distance of 500 metres, a thief starts running at a speed of 10 km/h. After noticing, the policeman chases immediately with a speed of 12 km/h, and the thief is caught. The distance run by the policeman is:
a.) 2.4 km
b.) 3 km
c.) 2.5 km
d.) 3.6 km
Answer: b)
Solution:
Relative speed of the policeman w.r.t the relative speed of the thief = 12 – 10 = 2 km/h
Time taken by the policeman to catch the thief = {(500/1000) ÷ 2} = (1/4) hours
So, distance covered by the policeman = 12 × (1/4) = 3 km
Hence, option b.
Topic: SSC_ Ratio and proportions
Level: 0
3.) ‘A’ varies jointly with ‘B’ and ‘C’. A = 6 when B = 3 and C = 2. Find A when B = 5 and C = 7
a.) 17.5
b.) 35
c.) 105
d.) 70
Answer: b)
Solution:
ATQ:
A = k × B × C {Where ‘k’ is a constant}
So, 6 = k × 3 × 2
Or, k = 1
Required value = A = 1 × 5 × 7 = 35
Hence, option b.
Topic: SSC_ Simplification
Level: 0
4.) Which of the following options has the greatest value?
a.) 20 + 4 + (−8) – 2 + 3 + 6
b.) (−22) + (−4 − 7)
c.) (−18) – 45 + (−3 − 2)
d.) (−99) + (−44) − 12
Answer: a)
Solution:
Option A: 20 + 4 + (−8) – 2 + 3 + 6
= 24 – 8 – 2 + 3 + 6 = 23
Option B: (−22) + (−4 − 7)
= -22 – 4 – 7 = –33
Option C: (−18) – 45 + (−3 − 2)
= -18 – 45 – 3 – 2 = –68
Option D: (−99) + (−44) − 12
= –99 – 44 – 12 = –155
So, option ‘A’ has the greatest value.
Hence, option a.
Topic: SSC_ Geometry and mensuration
Level: 0
Attempt SSC CHSL Tier I 2023 Free Mock Test
5.) In ΔABC, if G is the centroid and AD is a median with length 9 cm, then the length of AG is:
a.) 1.8 cm
b.) 5 cm
c.) 7 cm
d.) 6 cm
Answer: d)
Solution:
In a triangle with median AD and centroid ‘G’, the centroid divided GD and AG in ratio 1:2.
So, length of AG = 9 × (2/3) = 6 cm
Hence, option d.
Topic: SSC_ Compound interest and simple interest
Level: 0
6.) A sum of ₹14,375, when invested at r% interest per year compounded annually, amounts to ₹16,767 after two years. What is the value of r?
a.) 9
b.) 7
c.) 8
d.) 6
Answer: c)
Solution:
ATQ;
16767 = 14375 × {1 + (r/100)}2
Or, {1 + (r/100)}2 = (729/625)
Or, 1 + (r/100) = (27/25)
Or, (r/100) = (27/25) – 1
Or, (r/100) = (2/25)
Or, r = (2/25) × 100 = 8
Hence, option c.
Topic: SSC_ DI
Sub – Topic: Pie chart
Level: 0
Attempt SSC CHSL Tier I 2023 Free Mock Test
7.) In a School of 200 students, the following charts represent the percentage of students involved in different sports.
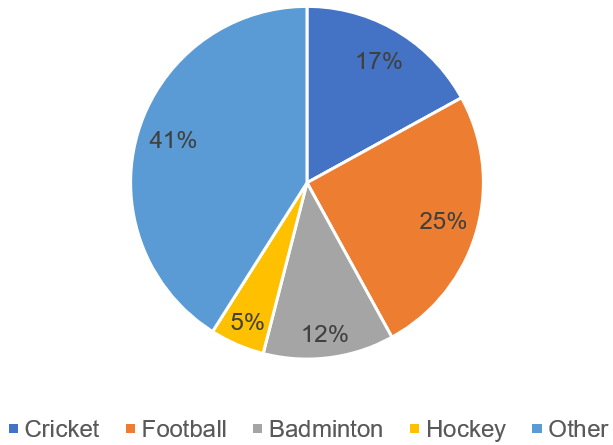
What is the number of students playing cricket?
a.) 34
b.) 24
c.) 83
d.) 17
Answer: a)
Solution:
Number of students playing cricket = 200 × (17/100) = 34
Hence, option a.
Topic: SSC_ Averages
Level: 0
8.) The average of 10 observations is 21. A new observation is included and the average of these 11 numbers is 1 less than the previous average. The 11th observation is ______.
a.) 21
b.) 11
c.) 10
d.) 12
Answer: c)
Solution:
Sum of first 10 observations = 10 × 21 = 210
Sum of 11 observations = 11 × 20 = 220
So, 11th observation = 220 – 210 = 10
Hence, option c.
Topic: SSC_ DI
Sub – Topic: Bar
Level: 0
Attempt SSC CHSL Tier I 2023 Free Mock Test
9.) Observe the graph and answer the question that follows:
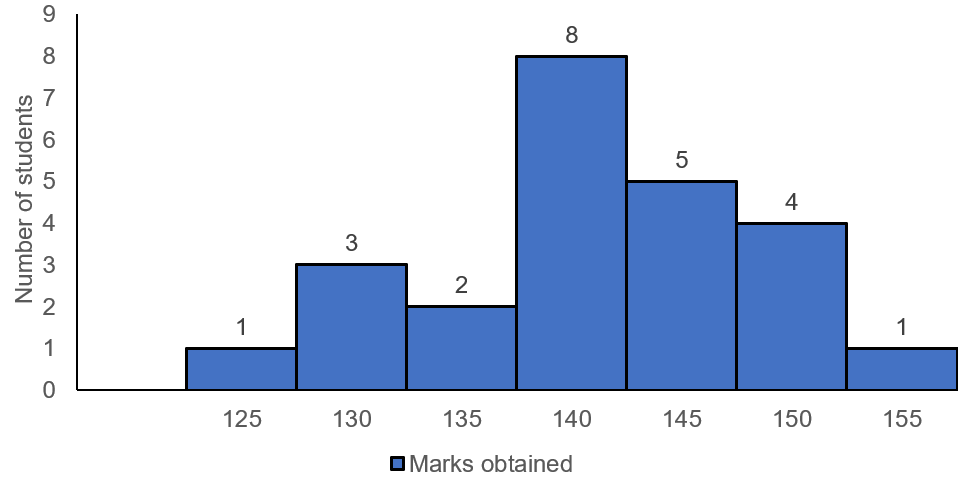
How many students obtained marks more than 130?
a.) 14
b.) 19
c.) 20
d.) 17
Answer: c)
Solution:
Required sum = 2 + 8 + 5 + 4 + 1 = 20
Hence, option c.
Topic: SSC_ Number system
Level: 0
10.) Which of the following is the least 6-digit number that is divisible by 93?
a.) 100068
b.) 100070
c.) 100075
d.) 100065
Answer: a)
Solution:
We know that 100000 ÷ 93 ~ 1075.26
So, required number must be 93 × 1076
So, least 6-digit number that is divisible by 93 = 93 × 1076 = 100068
Hence, option a.
Topic: SSC_ Ratio and proportions
Level: 0
Attempt SSC CHSL Tier I 2023 Free Mock Test
11.) A number ‘x’ is three times another number ‘y’. If the sum of both the numbers is 20, then x and y, respectively, are:
a.) 8 and 12
b.) 15 and 5
c.) 5 and 15
d.) 2 and 18
Answer: b)
Solution:
ATQ;
x = 3y
So, x + y = 3y + y = 20
Or, 4y = 20
So, y = 5
So, x = 20 – 5 = 15
Therefore, ‘x’ and ‘y’ are 15 and 5, respectively
Hence, option b.
Topic: SSC_ Percentage, profit and loss
Level: 0
Attempt SSC CHSL Tier I 2023 Free Mock Test
12.) A person could save 20% of his income. A year later, his income increased by 25% but he could save the same amount only as before. By what percentage has his expenditure increased?
a.) 27.5%
b.) 32.5%
c.) 31.25%
d.) 29.75%
Answer: c)
Solution:
Let the initial income of the person be Rs. 100x
So, amount saved = 100x × 0.2 = Rs. 20x
So, original expenditure = 100x – 20x = Rs. 80x
New income = 100x × 1.25 = Rs. 125x
So, new expenditure = 125x – 20x = Rs. 105x
Increase in expenditure = 105x – 80x = Rs. 25x
So, required percentage = (25x/80x) × 100 = 31.25%
Hence, option c.
Topic: SSC_Percentages, profit and loss
Level: 0
13.) The marked price of a table fan is Rs. 3750 and is available to the retailer at a discount of 20%. At what price should the retailer sell it to earn a profit of 15%?
a.) Rs. 3250
b.) Rs. 3500
c.) Rs. 3700
d.) Rs. 3450
Answer: d
Solution:
Price at which the retailer purchases the table fan = 3750 × 0.8 = Rs. 3000
So, price at which the retailer must sell the fan to earn a profit of 15% = 3000 × 1.15 = Rs. 3450
Hence, option d.
Topic: Time, speed and distance
Level: 0
14.) Ravi drove for 4 hours at a speed of 70 miles per hour and for 2 hours at a speed of 40 miles per hour. What was his average speed (in miles per hour) for the whole journey?
a.) 60 miles per hour
b.) 45 miles per hour
c.) 55 miles per hour
d.) 65 miles per hour
Answer: a
Solution:
Total distance travelled by Ravi = 4 × 70 + 2 × 40 = 360 miles
Total time taken for travel = 4 + 2 = 6 hours
So, average speed = total distance covered ÷ total time taken = (360/6) = 60 miles per hour
Hence, option a.
Topic: Algebra
Level: 0
Attempt SSC CHSL Tier I 2023 Free Mock Test
15.) What is the product of (x + a) and (x + b)?
a.) x2 + (a + b)x + ab
b.) x2 + (a – b)x + ab
c.) x2 + (a + b)x – ab
d.) x2 + (a – b)x – ab
Answer: a
Solution:
(x + a) × (x + b) = x2 + ax + bx + ab
= x2 + (a + b)x + ab
Hence, option a.
Topic: SSC_Mensuration
Level: 0
16. What is the height of a solid right circular cylinder whose radius is 3 cm and total surface area is 60π cm2.
a.) 4.2 cm
b.) 5 cm
c.) 7 cm
d.) 9 cm
Answer: c
Solution:
Total surface area of a cylinder = 2 × π × radius × (height + radius)
= 2 × π × 3 × (height + 3)
Or, 6π(height + 3) = 60π
So, height + 3 = 60π ÷ 6π = 10
So, height of the cylinder = 10 – 3 = 7 cm
Hence, option c.
Topic: SSC_Time and work
Level: 0
Attempt SSC CHSL Tier I 2023 Free Mock Test
17.) 45 people can repair a road in 10 days working 6 hours a day. In how many days can 30 people working 6 hours a day complete the same work?
a.) 12
b.) 10
c.) 18
d.) 15
Answer: d
Solution:
Let the hourly efficiency of 1 person be ‘x’ units/hour
Then, total work = 45x × 10 × 6 = 2700x
So, number of days taken by 30 people working 6 hours a day to complete the same work
= 2700x ÷ (30x × 6) = 15 days
Hence, option d.
Topic: SSC_Mensuration
Level: 0
18.) What is the area (in cm2) of an equilateral triangle of side 20 cm.
a.) 100√3 cm2
b.) 200 cm2
c.) 100 cm2
d.) 100√2 cm2
Answer: a
Solution:
For an equilateral triangle with side length of ‘a’ units
Area of the triangle = (√3/4)a2
= (√3/4) × 202 = 100√3 cm2
Hence, option a.
Topic: SSC_Percentages profit and loss
Level: 0
Attempt SSC CHSL Tier I 2023 Free Mock Test
19.) Under a discount scheme, a dozen pair of gloves quoted at Rs. 200 is available at a discount of 20%. How many pairs of gloves can be bought for Rs. 320?
a.) 15
b.) 20
c.) 18
d.) 24
Answer: d
Solution:
Selling price of 1 dozen pair of gloves = 200 × 0.8 = Rs. 160
So, number of dozens of pairs of gloves that can be bought for Rs. 320 = 320 ÷ 160 = 2
So, number of pairs of gloves that can be bought for Rs. 320 = 2 × 12 = 24
Hence, option d.
Topic: SSC_Trignometry
Level: 0
20.) The value of sin 73o + cos 137o is:
a.) sin 13o
b.) cos 13o
c.) cos 18o
d.) sin 18o
Answer: a
Solution:
sin 73o + cos 137o = sin 73o + cos (90 + 47)o
= sin 73o – sin 47o
We know that, sin A – sin B = 2cos{(A+ B)/2} sin{(A – B)/2}
So, sin 73o – sin 47o = 2cos{(73 + 47)/2} sin{(73 – 47)/2}
= 2cos 60o sin 13o
= 2 × (1/2) × sin 13o
= sin 13o
Hence, option a.
Topic: SSC_Mensuration
Level: 0
Attempt SSC CHSL Tier I 2023 Free Mock Test
21. The diameter of the base of a right circular cylinder in 12 cm and the height of the cylinder is 2.45 times the radius of its base. Find the curved surface area of the cylinder.
a.) 550.4 cm2
b.) 548.8 cm2
c.) 578.2 cm2
d.) 554.4 cm2
Answer: 4
Solution:
Radius of the given cylinder = diameter ÷ 2 = 12 ÷ 2 = 6 cm
So, height of the cylinder = 6 × 2.45 = 14.7 cm
Curved surface area of the cylinder = 2 × π × radius × height
= 2 × (22/7) × 6 × 14.7
= 554.4 cm2
Hence, option d.
Topic: SSC_Algebra
Level: 0
22.) If x + y + z = 10, x2 + y2 + z2 = 30, then the value of x3 + y3 + z3 – 3xyz is?
a.) -25
b.) -50
c.) -40
d.) -10
Answer: 2
Solution:
(x + y + z)2 = 102 = x2 + y2 + z2 + 2(xy + yz + zx)
So, 2(xy + yz + zx) = 100 – 30 = 70
So, (xy + yz + zx) = (70/2) = 35
x3 + y3 + z3 – 3xyz = (x + y + z)(x2 + y2 + z2 – xy – yz – zx)
= 10 × (30 – 35)
= 10 × – 5
= – 50
Hence, option b.
Topic: SSC_Percentages, profit and loss
Level: 0
Attempt SSC CHSL Tier I 2023 Free Mock Test
23.) A pen passing through 2 hands is finally sold at a profit of 44% of the original cost price. If the first dealer makes a profit of 20%, then the profit made by the second dealer is?
a.) 36%
b.) 24%
c.) 20%
d.) 27%
Answer: c
Solution:
Let the original cost price of the article be Rs. 100x
Then, price at which first dealer sold the article = 100x × 1.2 = Rs. 120x
Final selling price = 100x × 1.44 = Rs. 144x
So, profit made by the second dealer = (144x – 120x) ÷ 120x × 100 = 20%
Hence, option c.
Topic: SSC_Averages
Level: 0
24. A shopkeeper purchases 20000 units of a product at Rs. 1 per unit, 15000 units at Rs. 1.15 per unit and 5000 units at Rs. 2 per unit. What is the weight average price of one unit of product bought? (correct to two decimal places)
a.) Rs. 1.36
b.) Rs. 1.28
c.) Rs. 1.18
d.) Rs. 1.22
Answer: c
Solution:
Total price of all products purchased = 20000 × 1 + 15000 × 1.15 + 5000 × 2
= Rs. 20000 + 17250 + 10000 = Rs. 47250
Total number of products purchased = 20000 + 15000 + 5000 = 40000
So, required average = 47250 ÷ 40000 ~ 1.18
Hence, option c.
Topic: SSC_DI
Sub-Topic: Table
Level: 0
Attempt SSC CHSL Tier I 2023 Free Mock Test
25. The table below shows the number of cakes sold by six different bakeries in a town on five different days of a particular week.
| Days/Bakery | Monday | Tuesday | Thursday | Saturday | Sunday |
| A | 222 | 255 | 215 | 250 | 266 |
| B | 205 | 275 | 314 | 295 | 260 |
| C | 245 | 266 | 305 | 195 | 235 |
| D | 221 | 230 | 185 | 300 | 280 |
| E | 312 | 325 | 298 | 272 | 254 |
| F | 175 | 205 | 255 | 240 | 308 |
What is the total number of cakes sold by bakery ‘D’ on Monday, Thursday and Sunday taken together?
a.) 686
b.) 712
c.) 672
d.) 654
Answer: a
Solution:
Required sum = 221 + 185 + 280 = 686
Hence, option a.
Attempt SSC CHSL Tier I 2023 Free Mock Test
This brings us to the end of the article. So, here are few SSC CHSL Quant Practice Questions Free Preparation based on latest exam patern. Keep preparing for the upcoming SSC CHSL 2023 Tier I exam.
SSC CHSL 2023 Notification Out – Exam Date, Vacancies, Exam Pattern
- Sign Up on Practicemock for Updated Current Affairs, Free Topic Tests and Free Mini Mocks
- Sign Up Here to Download Free Study Material
Free Mock Tests for the Upcoming Exams
- IBPS PO Free Mock Test
- RBI Grade B Free Mock Test
- IBPS SO Free Mock Test
- NABARD Grade A Free Mock Test
- SSC CGL Free Mock Test
- IBPS Clerk Free Mock Test
- IBPS RRB PO Free Mock Test
- IBPS RRB Clerk Free Mock Test
- RRB NTPC Free Mock Test
- SSC MTS Free Mock Test
- SSC Strenographer Free Mock Test
- GATE Mechanical Free Mock Test
- GATE Civil Free Mock Test
- RRB ALP Free Mock Test
- SSC CPO Free Mock Test
- AFCAT Free Mock Test
- SEBI Grade A Free Mock Test
- IFSCA Grade A Free Mock Test
- RRB JE Free Mock Test
- Free Banking Live Test
- Free SSC Live Test



